转载

如何用Canvas画一个正多边形

本文由 BarryZhang 原创，同时首发于barryzhang.com，简书，非商业转载请注明作者和原文链接。

场景

给定一个指定的正方形的区域，要求在该区域内画一个正N边形（正三角形、正方形、正五边形……）

public static void drawPolygon (RectF rect, Canvas canvas, Paint p, int n) {
    // draw……
}

分析

要用到一些三角函数的知识，于是我画了一幅灵魂画作:ghost:：

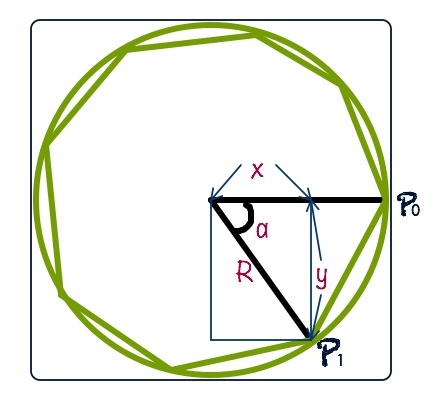
分析：

计算出每个顶点的坐标，然后把它们连起来，就是一个正多边形啦~
圆心角a的度数为 360/n ，弧度计算为 2π/n 。
如果把圆心的坐标为(0,0)，那么顶点P1的坐标为 [X1=cos(a),Y1=sin(a)] 。
以此类推，顶点Pn坐标为 [Xn=cos(a*n),Yn=sin(a*n)] 。
圆心的实际坐标是外接矩形的中心： [Ox=(rect.right+rect.left)/2 , Oy=(rect.top+rect.bottom)/2] 。
所以Pn的实际坐标是 [Xn+Ox,Yn+Oy] 。
把P0-P1…Pn连起来就是我们要的结果了。
Java中可以使用 Path 来保存路径，最后使用 canvas.drawPath 来绘制出来。

实现

简化的伪代码：

float a = 2π / n ; // 角度
Path path = new Path();
for( int i = 0; i < = n; i++ ){
    float x = R * cos(a * i); 
    float y = R * sin(a * i);
    if (i = 0){
        path.moveTo(x,y); // 移动到第一个顶点   
    }else{
        path.lineTo(x,y); //    
    }
}
drawPath(path);

Java代码最终的完整代码，可以直接拿去用：

public static void drawPolygon (RectF rect, Canvas canvas, Paint paintByLevel, int number) {
    if(number < 3) {
        return;
    }
    float r = (rect.right - rect.left) / 2;
    float mX = (rect.right + rect.left) / 2;
    float my = (rect.top + rect.bottom) / 2;
    Path path = new Path();
    for (int i = 0; i <= number; i++) {
        // - 0.5 : Turn 90 ° counterclockwise
        float alpha = Double.valueOf(((2f / number) * i - 0.5) * Math.PI).floatValue();
        float nextX = mX + Double.valueOf(r * Math.cos(alpha)).floatValue();
        float nextY = my + Double.valueOf(r * Math.sin(alpha)).floatValue();
        if (i == 0) {
            path.moveTo(nextX, nextY);
        } else {
            path.lineTo(nextX, nextY);
        }
    }
    canvas.drawPath(path, paintByLevel);
}